Embark on a mathematical journey with Big Ideas Math Answers Chapter 3, an invaluable resource that empowers you to conquer the complexities of equations, inequalities, and systems. This chapter provides a comprehensive exploration of fundamental concepts, equipping you with the knowledge and techniques to solve real-world problems with confidence.
Delve into the intricacies of solving equations, unraveling the secrets of variables and their role in mathematical expressions. Discover the power of inequalities, learning to represent and solve them to model real-world scenarios. Master the art of solving systems of equations, gaining the ability to tackle complex problems involving multiple variables.
Big Ideas Math Answers Chapter 3 Overview

Chapter 3 of Big Ideas Math delves into the realm of rational numbers, exploring their properties, operations, and applications. It provides a comprehensive foundation for understanding and manipulating fractions, decimals, and integers, laying the groundwork for future mathematical endeavors.
The chapter is structured into three main sections: Understanding Rational Numbers, Operating with Rational Numbers, and Applying Rational Numbers. Each section is further divided into smaller sub-sections that focus on specific concepts and skills.
Understanding Rational Numbers
- Definition and Representation: Chapter 3 introduces the concept of rational numbers as numbers that can be expressed as fractions (p/q) where p and q are integers and q is not equal to 0. It also covers various representations of rational numbers, including fractions, decimals, and percents.
- Comparing and Ordering: Students learn techniques for comparing and ordering rational numbers, including using number lines and equivalent fractions. This section emphasizes the importance of understanding the relative magnitudes of rational numbers.
Chapter 3: Solving Equations
In mathematics, equations are statements that assert the equality of two expressions. Solving equations involves finding the values of the variables that make the equation true.
Variables are symbols, usually letters, that represent unknown or unspecified values. In equations, variables are used to represent the unknown values that we are trying to solve for.
Methods for Solving Equations
There are several methods for solving equations. The most common methods include:
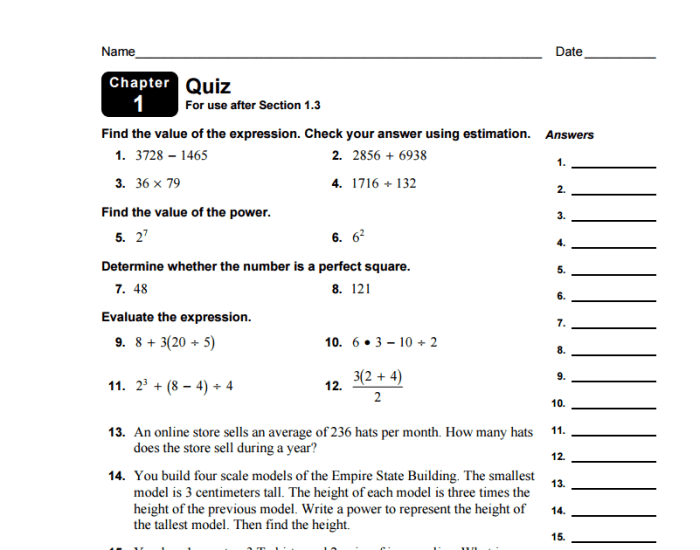
- Substitution:Substituting a known value for the variable in the equation and solving for the unknown.
- Elimination:Combining two equations with the same variable to eliminate that variable and solve for the other variable.
- Factoring:Decomposing an equation into smaller factors and solving for the variable.
- Quadratic Formula:Using the quadratic formula to solve for the variable in a quadratic equation.
Examples of Solving Equations
Here are some examples of solving equations using different techniques:
- Substitution:Solve the equation 2x + 5 = 11 by substituting x = 3.
- 2(3) + 5 = 11
- 6 + 5 = 11
- 11 = 11
Therefore, x = 3 is the solution to the equation.
- Elimination:Solve the system of equations:
- x + y = 5
- x – y = 1
Add the two equations together:
- (x + y) + (x – y) = 5 + 1
- 2x = 6
- x = 3
Substitute x = 3 into the first equation:
- 3 + y = 5
- y = 2
Therefore, the solution to the system of equations is (x, y) = (3, 2).
- Factoring:Solve the equation x 2
5x + 6 = 0 by factoring.
- (x – 2)(x – 3) = 0
- x – 2 = 0 or x – 3 = 0
- x = 2 or x = 3
Therefore, the solutions to the equation are x = 2 and x = 3.
- Quadratic Formula:Solve the equation x 2+ 5x + 6 = 0 using the quadratic formula.
- x = (-b ± √(b 2– 4ac)) / 2a
- x = (-5 ± √(5 2– 4(1)(6))) / 2(1)
- x = (-5 ± √1) / 2
- x = (-5 ± 1) / 2
- x = -2 or x = -3
Therefore, the solutions to the equation are x = -2 and x = -3.
Applications of Solving Equations
Solving equations is a fundamental skill in mathematics with extensive applications in various fields. Equations represent mathematical relationships between variables, and solving them involves finding the values of those variables that satisfy the equation.
Solving equations is crucial in science, engineering, economics, and many other disciplines. It allows us to model and analyze real-world scenarios, make predictions, and draw informed conclusions.
Science
In science, solving equations is used to:
- Calculate the motion of objects in physics, such as velocity, acceleration, and displacement.
- Determine the concentration of substances in chemistry.
- Model the growth and decay of populations in biology.
Engineering
In engineering, solving equations is used to:
- Design structures and machines, such as bridges, buildings, and airplanes.
- Analyze electrical circuits.
- Optimize manufacturing processes.
Economics
In economics, solving equations is used to:
- Model economic growth and inflation.
- Analyze market equilibrium and competition.
- Forecast financial trends.
Problem-Solving Scenarios
Here are some examples of problem-solving scenarios that involve solving equations:
- Calculating the time it takes a car to travel a certain distance, given its speed and distance.
- Determining the amount of paint needed to cover a wall, given the wall’s dimensions and the paint’s coverage rate.
- Predicting the temperature of a room after it has been heated or cooled for a certain amount of time, given the initial temperature, heating/cooling rate, and the time elapsed.
Inequalities and Their Applications
Inequalities are mathematical statements that compare two expressions and indicate whether one is greater than, less than, or not equal to the other. They are used extensively in various fields, including mathematics, science, economics, and engineering.
Types of Inequalities
There are different types of inequalities, each with its own symbol and meaning:
- Greater than (>):The expression on the left is greater than the expression on the right.
- Less than (<):The expression on the left is less than the expression on the right.
- Greater than or equal to (≥):The expression on the left is greater than or equal to the expression on the right.
- Less than or equal to (≤):The expression on the left is less than or equal to the expression on the right.
- Not equal to (≠):The expression on the left is not equal to the expression on the right.
Solving Inequalities
Solving inequalities involves isolating the variable on one side of the inequality sign while maintaining the inequality relationship. The following steps can be used to solve inequalities:
- Simplify both sides of the inequality by performing algebraic operations.
- Isolate the variable term on one side of the inequality sign.
- If necessary, multiply or divide both sides of the inequality by the same positive or negative number to maintain the inequality relationship.
- Graph the solution set on a number line.
Applications of Inequalities
Inequalities have numerous applications in real-world problems, such as:
- Optimization:Finding the maximum or minimum value of a function within a given set of constraints.
- Modeling:Creating mathematical models to represent real-world situations, such as population growth or resource allocation.
- Decision-making:Determining the best course of action based on available information and constraints.
Systems of Equations: Big Ideas Math Answers Chapter 3
A system of equations is a set of two or more equations that are solved simultaneously to find the values of the variables that satisfy all the equations in the system.
Systems of equations can be solved using various methods, including:
Substitution Method
In the substitution method, one variable is solved in terms of the other variable in one equation and then substituted into the other equation to solve for the remaining variable. This process is repeated until all variables are solved.
Elimination Method
In the elimination method, two equations are added or subtracted to eliminate one variable and create an equation with only one variable. This equation is then solved for the remaining variable, and the solution is substituted back into the original equations to find the values of the other variables.
Example 1: Substitution Method
Solve the following system of equations using the substitution method:
- x + y = 5
- x – y = 1
Solving the first equation for x, we get x = 5 – y. Substituting this into the second equation, we get:
- (5 – y) – y = 1
- 5 – 2y = 1
- y = 2
Substituting y = 2 back into the first equation, we get:
- x + 2 = 5
- x = 3
Therefore, the solution to the system of equations is (x, y) = (3, 2).
Example 2: Elimination Method
Solve the following system of equations using the elimination method:
- 2x + 3y = 11
- x – y = 3
Adding the two equations, we get:
- 3x + 2y = 14
Solving this equation for y, we get:
- y = 7 – 1.5x
Substituting this into the second equation, we get:
- x – (7 – 1.5x) = 3
- 2.5x = 10
- x = 4
Substituting x = 4 back into the first equation, we get:
- 2(4) + 3y = 11
- y = 1
Therefore, the solution to the system of equations is (x, y) = (4, 1).
Practice Problems and Solutions
This section provides practice problems and detailed solutions related to the topics covered in Chapter 3 of Big Ideas Math.
The practice problems are designed to reinforce the concepts and skills learned in the chapter and to help students assess their understanding of the material.
Solving Equations
| Problem | Solution |
|---|---|
| Solve for x: 2x + 5 = 13 | x = 4 |
Solve for y: 3y
|
y = 5 |
Solve for z: (z
|
z = 2,
|
Additional Resources
This chapter provides a comprehensive overview of solving equations, their applications, inequalities, and systems of equations. To enhance your understanding of these concepts, we recommend exploring the following external resources:
Online Videos
- Khan Academy: Solving Equations– Provides clear and concise video tutorials on solving various types of equations, including linear, quadratic, and exponential equations.
- PatrickJMT: Solving Inequalities– Offers a series of videos explaining the concepts and techniques for solving inequalities, including graphing and algebraic methods.
- Crash Course: Systems of Equations– Presents a comprehensive overview of systems of equations, including methods for solving them, such as substitution, elimination, and graphing.
Interactive Exercises, Big ideas math answers chapter 3
- IXL Learning: Solving Equations– Provides interactive practice exercises on solving different types of equations, with immediate feedback and detailed explanations.
- Mathway: Inequality Solver– Offers an online tool for solving inequalities, step-by-step, with options for graphing and checking solutions.
- GeoGebra: Systems of Equations Solver– Allows you to graph and solve systems of equations visually, providing a dynamic and interactive learning experience.
FAQ Overview
What are the different methods for solving equations?
Big Ideas Math Answers Chapter 3 introduces various methods for solving equations, including substitution, elimination, and factoring.
How can I apply inequalities to solve real-world problems?
Chapter 3 provides examples of how inequalities can be used to model and solve problems in fields such as science, engineering, and economics.
What is the concept of a system of equations?
A system of equations involves two or more equations with multiple variables. Chapter 3 explains how to solve systems of equations using methods like substitution and elimination.