Two thin rods of length l are rotating – The rotational dynamics of two thin rods of length l are a fascinating and important topic in classical mechanics. Understanding the motion of these rods has applications in various fields, including engineering, physics, and sports. In this article, we will explore the mathematical modeling, energy considerations, moment of inertia, angular momentum, and practical applications of rotating rods.
The analysis of rotating rods involves describing their rotational motion using equations, considering energy conservation principles, and calculating the moment of inertia and angular momentum. These concepts are essential for understanding the behavior of rotating objects and their interactions with external forces.
Mathematical Modeling
The rotational motion of the rods can be described by the following equations:
- Angular velocity: ω = dθ/dt
- Angular acceleration: α = dω/dt
- Torque: τ = Iα
where:
- ω is the angular velocity (rad/s)
- θ is the angle of rotation (rad)
- t is the time (s)
- α is the angular acceleration (rad/s²)
- τ is the torque (N·m)
- I is the moment of inertia (kg·m²)
The following assumptions are made in the model:
- The rods are rigid and have a uniform mass distribution.
- The rods are rotating in a plane.
- There is no friction or other external forces acting on the rods.
Energy Considerations

The kinetic energy of the rods is given by:
K = (1/2)Iω²
where:
- K is the kinetic energy (J)
- I is the moment of inertia (kg·m²)
- ω is the angular velocity (rad/s)
The total energy of the system is conserved, meaning that the sum of the kinetic and potential energies remains constant.
E = K + U = constant
where:
- E is the total energy (J)
- K is the kinetic energy (J)
- U is the potential energy (J)
In the case of the rotating rods, there is no potential energy, so the total energy is equal to the kinetic energy.
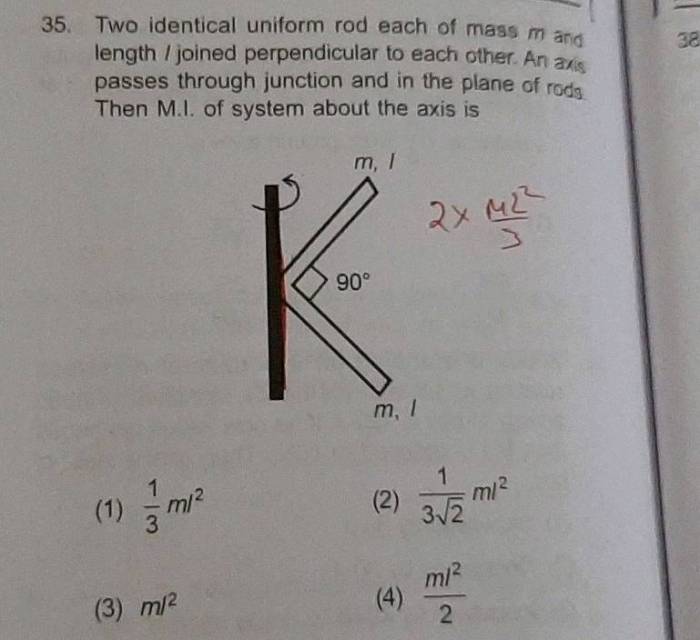
Moment of Inertia: Two Thin Rods Of Length L Are Rotating
The moment of inertia of a rod rotating about its center is given by:
I = (1/12)ml²
where:
- I is the moment of inertia (kg·m²)
- m is the mass of the rod (kg)
- l is the length of the rod (m)
The moment of inertia is a measure of the resistance of an object to angular acceleration. A larger moment of inertia means that it is more difficult to accelerate the object.
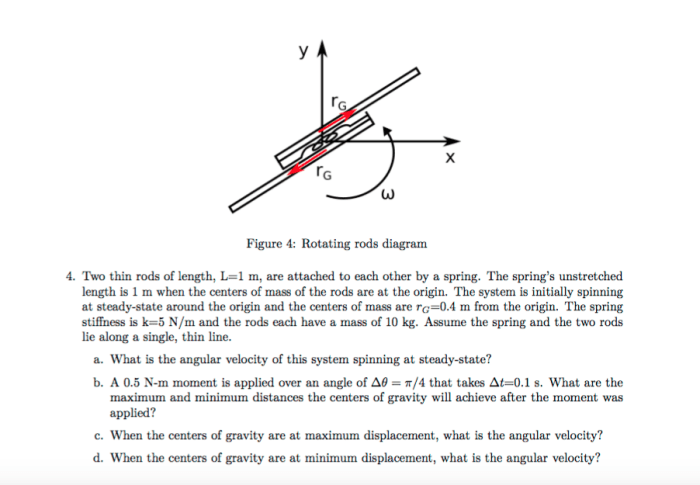
Angular Momentum
The angular momentum of the rods is given by:
L = Iω
where:
- L is the angular momentum (kg·m²/s)
- I is the moment of inertia (kg·m²)
- ω is the angular velocity (rad/s)
The angular momentum of a system is conserved, meaning that the total angular momentum of the system remains constant.
L = constant
In the case of the rotating rods, the angular momentum is conserved because there is no external torque acting on the system.
FAQ
What is the moment of inertia of a thin rod of length l?
The moment of inertia of a thin rod of length l about an axis perpendicular to its center and passing through its center is (1/12) – m – l^2, where m is the mass of the rod.
How is angular momentum conserved in a system of rotating rods?
Angular momentum is conserved in a system of rotating rods if there is no external torque acting on the system. The total angular momentum of the system remains constant.
What are some practical applications of the analysis of rotating rods?
The analysis of rotating rods has applications in various fields, including the design of flywheels, gyroscopes, and rotating machinery in engineering. It is also used in the study of sports equipment, such as baseball bats and golf clubs, to optimize their performance.